GATE Mechanical 2021 Set 2 | GA Question: 9

Question

Consider a square sheet of side $1$ unit. The sheet is first folded along the main diagonal. This is followed by a fold along its line of symmetry. The resulting folded shape is again folded along its line of symmetry. The area of each face of the final folded shape, in square units, equal to _________

• A. $\frac{1}{4}$

• A. $\frac{1}{8}$

100. $\frac{1}{16}$

500. $\frac{1}{32}$

Answer 1

Given that a square sheet has a side length of $1$ unit.

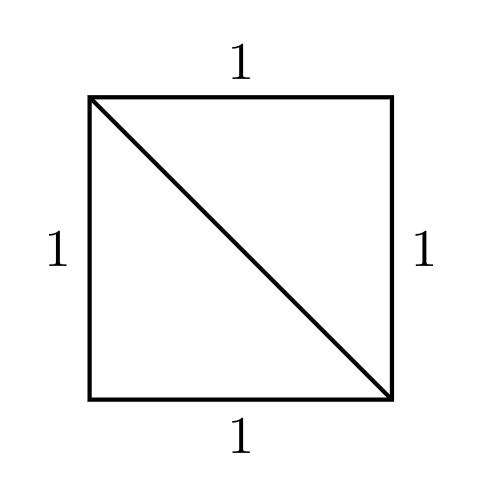
Now, the sheet is first folded along the main diagonal. 

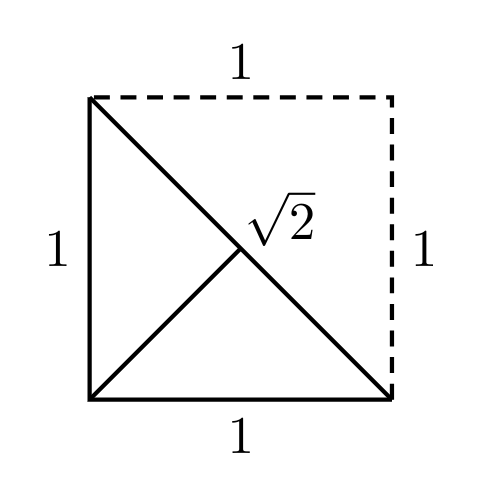
Again, this is followed by a fold along its line of symmetry. And this operation is performed two times.

We get the final folded sheet.

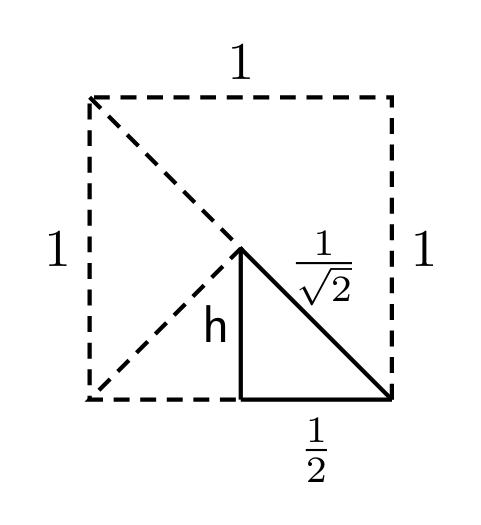
Let the height of the triangle be $h$ units.

Using the Pythagorean theorem, we get.

$\left(\frac{1}{\sqrt{2}}\right)^{2} = h^{2} + \left(\frac{1}{2}\right)^{2}$

$\implies \frac{1}{2} = h^{2} + \frac{1}{4}$

$\implies h^{2} = \frac{1}{2} – \frac{1}{4}$

$\implies h^{2} = \frac{2 – 1 }{4} = \frac{1}{4}$

$\implies h = \frac{1}{2}$

Now, the area of triangle $\Delta = \frac{1}{2} \times \text{Base} \times \text{Height}$

$\implies \Delta = \frac{1}{2} \times \frac{1}{2} \times \frac{1}{2} = \frac{1}{8}\;\text{unit}^{2}.$

$\textbf{Short Method:}$ Area follows the symmetry.

• Initially the area of square $ = 1$
• Folded first time, then the area of square $ = \frac{1}{2}$
• Folded second time, then the area of square $ = \frac{1}{4}$
• Folded third time, then the area of square $ = \frac{1}{8}$

So, the correct answer is $(B).$